放物線の定義と方程式
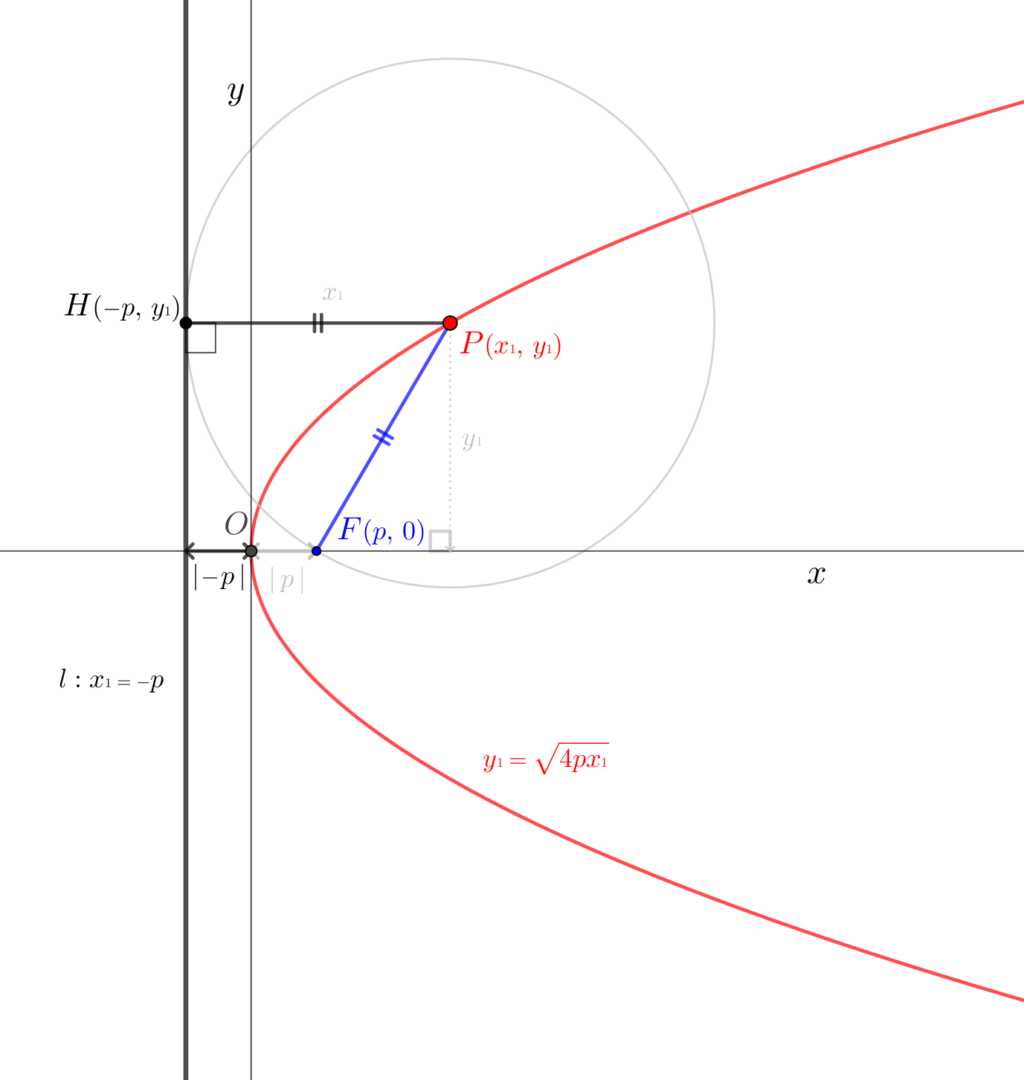
上図\(1\)から、放物線とは、直線 \(l\)\((\)準線という\()\) と、その上にない任意の一点 \(\color{red}P\) を中心点とする円周上の点 \(\color{blue}F\) をとるとき\((\)ただし\(H\)\(\neq\)\(\color{blue}F\)。点\(H\)は点\(\color{red}P\)から\(l\)に垂線をおろした交点。\(\)\()\)、 \(\color{red}P\)から焦点 \(\color{blue}F\) への距離 \(\overline{{\color{red}P}{\color{#blue}F}}\) と等しい距離 \(\overline{{\color{red}P}{\color{#000}H}}\) を持つような準線 \(l\) 上の点 \(H\) が存在するようなものの 軌跡 として定義される平面曲線 である。
赤い曲線\((\)つまり放物線\()\)を軌道とする点を \(\color{red}P(x{\tiny 1},\,y{\tiny 1})\)、焦点を \(\color{blue}F(p,\,0)\)、準線\(l\)の式を \(y{\tiny 1}{\small\;=-}p\) とすると、
上図の放物線の定義式は\(\hspace{10pt}\)\(\overline{{\color{red}P}{\color{blue}F}}{\small\;=\;}\overline{{\color{red}P}{H}}\) より
\(\sqrt{(x{\tiny 1}{\small\,-\,}p)^{2}{\small\,+\,}y{\tiny 1}^{2}}\)\({\small\;=\;}|x{\tiny 1}{\small\,-\,}({\small -}p)|\)\(\hspace{10pt}{\small\Rightarrow}\hspace{10pt}\)\((x{\tiny 1}{\small\,-\,}p)^{2}{\small\,+\,}y{\tiny 1}^{2}\)\({\small\;=\;}x{\tiny 1}^{2}{\small\,+\,}2\,x{\tiny 1}y{\tiny 1}{\small\,+\,}y{\tiny 1}^{2}\)\(\hspace{10pt}{\small\Rightarrow}\hspace{10pt}\)\(y{\tiny 1}^{2}{\small\;=\;}{4\,px{\tiny 1}}\)\(\hspace{10pt}{\small\Rightarrow}\hspace{10pt}\)\(y{\tiny 1}{\small\;=\;}\sqrt{4\,px{\tiny 1}}\)
となる\((\)以下で解説\()\)。
\(\overline{\small{\color{red}P}{\color{blue}F}}\) の式 \(\small\sqrt{(x{\tiny 1}{\small\,-\,}p)^{2}{\small\,+\,}y{\tiny 1}^{2}}\) は三角関数から、\(\href{https://showanojoe.com/template-math/trigonometric-function/three-squares-theorem/}{\color{teal}\textsf{三平方の定理}}\)を用いた\(2\)点\(\small{\color{red}P}\)、\(\small{\color{blue}F}\) 間の距離による。
\(\overline{\small{\color{red}P}{H}}\) は\(\href{https://showanojoe.com/template-math/supplementary-material/straight-lines-and-equations/#3}{\color{teal}\textsf{点と直線の距離}}\)から公式 \(d\)\((\)\(2\)点間の距離を表す記号\()\)\({\small\;=\;}\)\({\small\displaystyle\frac{|ax{\tiny 2}{\small\,+\,}by{\tiny 2}{\small\,+\,}c|}{\sqrt{a^{2}{\small\,+\,}b^{2}}}}\) に 準線\(l\)の式 \(|x{\tiny 1}{\small\;=-}p|\) を直線の式の一般形に変形した \(x{\tiny 1}{\small +}p{\small\,=\,}0\) の左辺の項を代入した式 \({\small\displaystyle\frac{|1x{\tiny 2}{\small\,+\,}0y{\tiny 2}{\small\,-\,}({\small -}p)|}{\sqrt{1^{2}{\small\,+\,}0^{2}}}}\)\(\hspace{10pt}{\small\Rightarrow}\hspace{10pt}\)\(|x{\tiny 1}{\small\;=-}({\small -}p)|\)。
